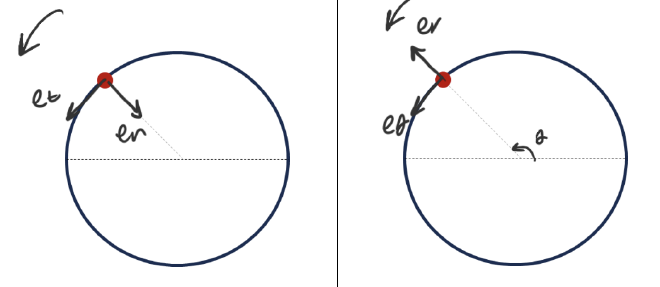
Position in R-Theta System
#

想要描述一个物体在 Coordinate 中的位置，可以通过

$$ \vec r=r\hat e_r $$

Velocity in R-Theta System
#

将 Position 求导就可以得到 Velocity，有

$$ \vec{v} = \frac{d\vec{r}}{dt} = \frac{d(r \hat{e}_r)}{dt} = \dot{r} \hat{e}_r + r \dot{\hat{e}}_r=\dot{r} \hat{e}_r + r \dot{\hat{e}}_r= \dot{r} \hat{e}r + r \dot{\theta} \hat{e}\theta $$

于是就得到了两个方向上的 Velocity

ex. Finding the velocity
#

A carousel is turning at the speed of 8 rpm. A child initially at 4m from the center walks toward the center at 2m/s. Find $\vec v$

$$ \vec{V} = \dot{r} \hat{e}r + r \dot{\theta} \hat{e}\theta = -2 \hat{e}r + (14)(0.84) \hat{e}\theta \quad \text{m/s} $$

Acceleration in R-Theta System
#

再次对于 velocity 求导得到

$$ \vec{a} = \frac{d\vec{v}}{dt} = \frac{d}{dt} (\dot{r} \hat{e}r + r \dot{\theta} \hat{e}\theta) = \ddot{r} \hat{e}r + \dot{r} \dot{\hat{e}}r + \dot{r} \dot{\theta} \hat{e}\theta + r \ddot{\theta} \hat{e}\theta + r \dot{\theta} \dot{\hat{e}}_\theta = \hat{e}r (\ddot{r} - r \dot{\theta}^2) + \hat{e}\theta (\ddot{\theta} + 2 \dot{r} \dot{\theta}) $$

$$ \Rightarrow a = \hat{e}r (\ddot{r} - r \dot{\theta}^2) + \hat{e}\theta (r\ddot{\theta} + 2 \dot{r} \dot{\theta}) $$

ex. Finding the Acceleration
#

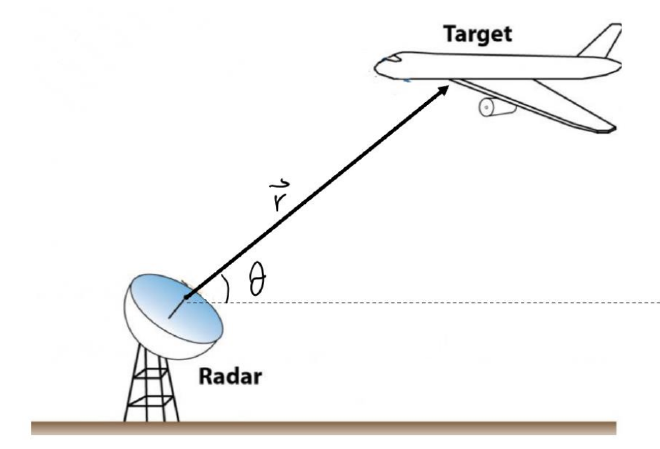
Extracted Problem Statement:

A shuttle is launched vertically and tracked by a radar station. At the instant when $\theta = 60^\circ$, $r = 9 km, $\ddot{r} = 21 \text{ m/s}^2$, and $\dot{\theta} = 0.02 \text{ s}^{-1}$, determine the acceleration vector $\vec{a}$

计算航天器的加速度 $\mathbf{a}$，给定数据如下：

![[DYN4.NormalandTangentialCoordinates-2.png]]

Special Case: Circular Motion
#

对于 Polar Coordinate 中的 Circular Motion，由于 $\dot r=\ddot r=0$ 很多公式都会被化简

N-T Coordinate
#

在 Circular Motion 中有

$$ v= ve_t $$

其本身就由 Tangential Direction 上的 Velocity 决定，不受影响

R-Theta Coordinate
#

对于一个 Polar Coordinate 中的 Circular Motion，有

$$ v=\dot{r} \hat{e}r + r \dot{\theta} \hat{e}\theta $$

带入 $\dot r=\ddot r=0$，得到

$$ v=r \dot{\theta} \hat{e}_\theta $$

Clockwise & Counter Clockwise
#

对于不同的运动方向，可以建立两个坐标系的关系
对于两个坐标系，其中一个特点就是 N-T Coordinate 中，Normal Force 是指向圆心的，而对于 R-Theta 来说，Radius 是从圆心指向物体 Postion 的，这直接导致了两个 Coordinate 下，有 $\vec a_n=-\vec a_r$
再者就是对于 R-Theta 中的 Angle 来说，Angle 的变化的永远以 Counter Clockwise 为正，但是 N-T 中不同转向的 Tangential Force 的正负不同，所以当 CW 时，有 $\vec a_t=-\vec a_\theta$，在 CCW 中则是 $\vec a_t=\vec a_\theta$